The Landau-Lifshitz-Gilbert equation

With the rapidly-increasing processing capability of modern computers, there has been a surge of interest in the field of computational micromagnetics, and indeed computer-based simulation in general. An important differential equation was derived by Landau and Lifshitz (1935).

The Landau-Lifshitz-Gilbert equation, briefly introduced in section 2.5, is a fundamental part of time-dependent computational micromagnetics. Different arrangements of this equation are used in calculations and simulations.The OOMMF simulation software (Donahue and Porter, 1999) uses the Landau and Lifshitz form:

$${{\mathrm{d}\ensuremath{\mathbf{M}}(\ensuremath{\mathbf{r}},{t})} \over{\mathrm{d}t}} = -\vert\bar\gamma\vert\ensuremath{\mathbf{M}}(\ensuremath{\mathbf{... ...uremath{\mathbf{H_{eff}}}(\ensuremath{\mathbf{M}}(\ensuremath{\mathbf{r}},{t}))$$

$$- {\vert\bar\gamma\vert\alpha \over M_s}\ensuremath{\mathbf{M}}(\... ...remath{\mathbf{H_{eff}}}(\ensuremath{\mathbf{M}}(\ensuremath{\mathbf{r}},{t})))$$ (2.36)

$\ensuremath{\mathbf{M}}$Magnetisation $\ensuremath{\mathbf{H}}_{\mathrm{eff}}$The effective magnetic field, a function of the total energy $\mathcal{E}$ $\alpha$The Landau and Lifshitz phenomenological damping parameter which is more commonly written as

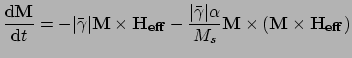$${{\mathrm{d}\ensuremath{\mathbf{M}}}\over{\mathrm{d}t}} = {-\vert... ...thbf{M}} \times (\ensuremath{\mathbf{M}} \times \ensuremath{\mathbf{H_{eff}}})}$$ (2.37)

where $\ensuremath{\mathbf{M}}$ is the magnetisation (i.e. the magnetic moment per unit volume), $\ensuremath{\mathbf{H_{eff}}}$ is the effective magnetic field, $\alpha$ is the Landau and Lifshitz phenomenological damping parameter (where $\bar\alpha$ from equation 2.34 is equivalent to $\vert\bar\gamma\vert\alpha$) and $\bar\gamma$ is the Landau and Lifshitz electron gyromagnetic ratio (the ratio of the magnetic dipole moment to the mechanical angular momentum of some system). If one assumes

$$\gamma = (1 + \alpha^2)\bar \gamma$$ (2.38)

then this can be shown to be mathematically equivalent to the Gilbert form (Gilbert, 1955)

$${{\mathrm{d}\ensuremath{\mathbf{M}}}\over{\mathrm{d}t}} = {-\vert... ...f{M}} \times {\mathrm{d}\ensuremath{\mathbf{M}} \over {\mathrm{d}t}} \right )}}$$ (2.39)